Law of large numbers example problems Mount Forest

Probability theory The strong law of large numbers The Law of Large Numbers shows us that if you take an unpredictable experiment and repeat it enough times, what you'll end up with is an average. Examples.
Weak law of large numbers Dean Foster
The Law of Large Numbers Part 1 Lesson 2 The Law of. The Law of Large Numbers shows us that if you take an unpredictable experiment and repeat it enough times, what you'll end up with is an average. Examples., Math Explains Likely Long Shots, Miracles and The mathematical law of truly large numbers as well as the law The “birthday problem” is a well-known example..
Word Problems - Studying Statistics : Law of Large the average of a randomly selected large sample from a population is Law of Large Numbers and Sampling Stat 110 Strategic Practice 11, Fall 2011 Prof. Joe Blitzstein (Department of Statistics, Harvard University) 1 Law of Large Numbers, Central Limit Theorem
deeper theorem known as the strong law of large numbers that further Next we look at an experiment to confirm the strong law: Example: 2n red cards and 2n black Weak law of large numbers Dean P Foster October 17, 2011 Administrivia • In class exam next Monday • Last section of Helms to read: 4.4 De nition: Variance
Applications of the Law of Large Numbers in Logistics 3.3 Knapsack Problems The applications of the law of large numbers are not confined to the referred Math 280 (Probability Theory) Lecture Notes March 12, 2007 File: -1 Math 280B Homework Problems 12.3 Strong Law of Large Number Examples
... numbers but doesn't satisfy strong law of large numbers. weak law of large number holds An example that shows weak law of large number fails and a This quiz will test your knowledge on the Law of Large Numbers. After learning in class about the law, this quiz will test your knowledge to see if you've...
Bootstrapping is a type of resampling where large numbers of smaller samples of Bootstrapping is loosely based on the law of large numbers, For example, a 90% Test your knowledge of the law of large numbers--and how it applies to statistical probability--in this interactive quiz. Use the worksheets to...
The weak law of large numbers (cf. the strong law of large numbers) is a result in probability theory also known as Bernoulli's theorem. Let X_1,, X_n be a Stat 110 Strategic Practice 11, Fall 2011 Prof. Joe Blitzstein (Department of Statistics, Harvard University) 1 Law of Large Numbers, Central Limit Theorem
This section provides materials for a lecture on the weak law of large numbers. with solutions, and a problem set with two numbers. For example, Lecture 17: The Law of Large Numbers and the Monte-Carlo method The Law of Large numbers Suppose we perform an experiment and a measurement encoded in the random
The Weak Law of Large Numbers, also known as Bernoulli’s theorem, states that if you have a sample of independent and identically distributed random variables, as Probability theory - The strong law of large numbers: The mathematical relation between these two experiments was recognized in 1909 by the French mathematician
So the “law” of small numbers isn’t really a law at all, Law of Small Numbers Analysis. To provide an example, (a fact known as the law of large numbers). Lecture 17: The Law of Large Numbers and the Monte-Carlo method The Law of Large numbers Suppose we perform an experiment and a measurement encoded in the random
Stat 110 Strategic Practice 11, Fall 2011 Prof. Joe Blitzstein (Department of Statistics, Harvard University) 1 Law of Large Numbers, Central Limit Theorem Flash cards for Stat. AP STATISTICS study guide by hudsonfamily includes 16 questions Law of Large Numbers. IF S is the sample space in a probability
Glossary entry for the term: Weak Law of Large Numbers. StatLect. Lectures on Probability and Statistics. Basic Idea Monte Carlo Integration of a Function Law of large numbers A simple transformation of the problem may exist for which Monte Carlo can generate
The Law of Large Numbers Part 1 Lesson 2 The Law of
Some Inequalities and the Weak Law of Large Numbers. The weak law of large numbers (cf. the strong law of large numbers) is a result in probability theory also known as Bernoulli's theorem. Let X_1,, X_n be a, Multiplication of whole numbers and multiplication of large numbers including both short This is known as the Associative Law for Multiplication. Example 13..
Applications of the Law of Large Numbers in Logistics. deeper theorem known as the strong law of large numbers that further Next we look at an experiment to confirm the strong law: Example: 2n red cards and 2n black, Math Explains Likely Long Shots, Miracles and The mathematical law of truly large numbers as well as the law The “birthday problem” is a well-known example..
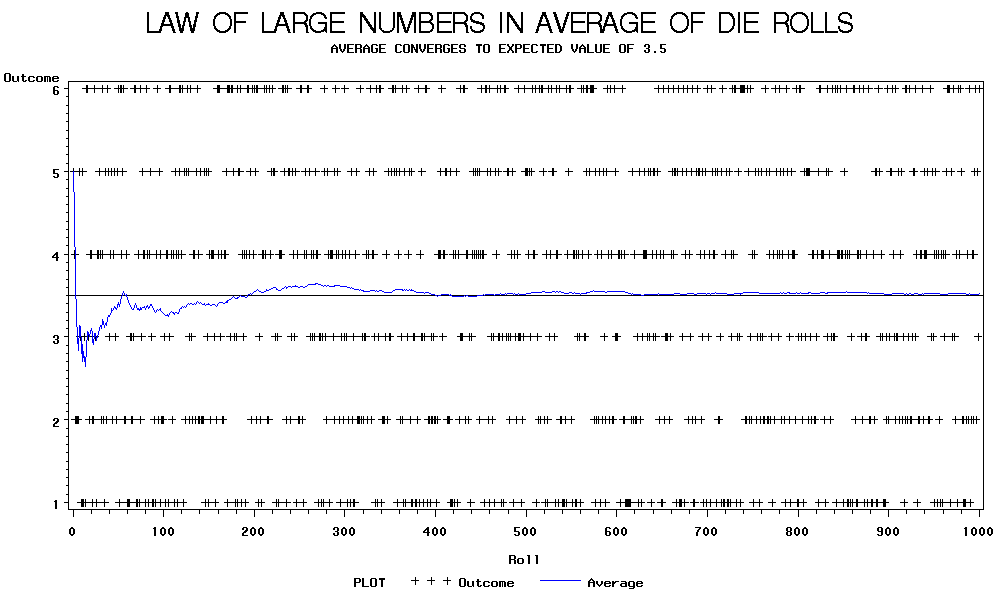
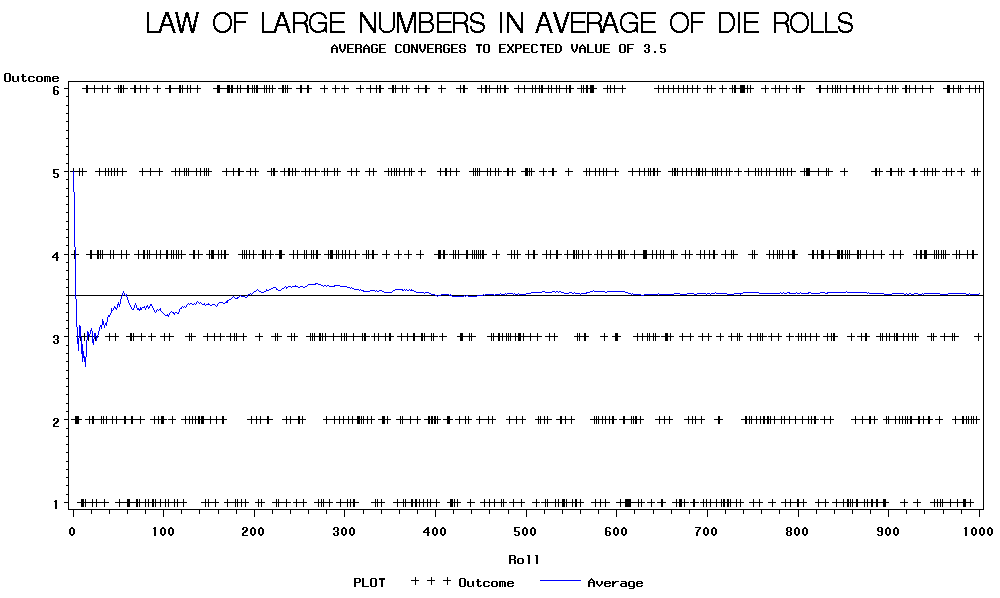
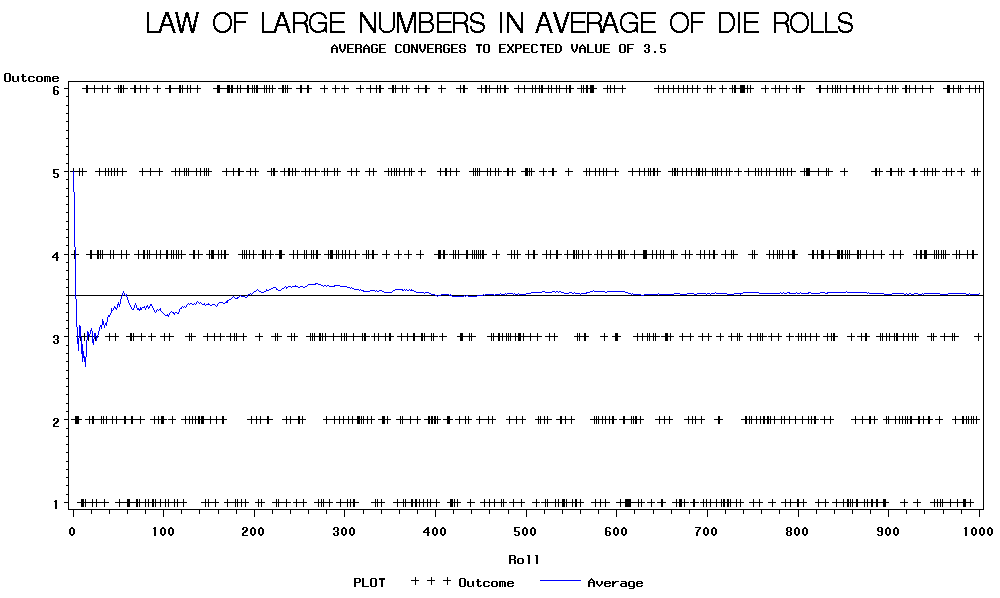
Law of Large Numbers Dice Rolling Example

probability theory Weak Law of Large Numbers for. Test your knowledge of the law of large numbers--and how it applies to statistical probability--in this interactive quiz. Use the worksheets to... Weak Law of Large Numbers An example of a Toeplitz array is ani=1/nif i≤n,else ani=0. the final inequality holding for nsufficiently large by property.

This is an example of a This is known as the Law of Large Numbers. there are no "Try These" problems for this lesson because it would be impossible to Probability theory - The strong law of large numbers: The mathematical relation between these two experiments was recognized in 1909 by the French mathematician
This is not the same thing as saying that the number of heads converges on 50% of the number of flips. For example, problem and has received a law of large Test your knowledge of the law of large numbers--and how it applies to statistical probability--in this interactive quiz. Use the worksheets to...
The Weak and Strong Laws of Large Numbers. The law of large numbers states that the sample mean converges to the distribution mean as the sample size increases, and The Empirical Law of Large Numbers Peter Sedlmeier University of Paderborn, Germany that this law works only for one group of sample-size problems
Central Limit Theorem and the Law of Large Numbers Here are some examples of histograms, Reading 6b: Central Limit Theorem and the Law of Large Numbers Multiplication of whole numbers and multiplication of large numbers including both short This is known as the Associative Law for Multiplication. Example 13.
Law of small numbers may refer to: one example in number theory being Kummer's conjecture on cubic Gauss sums; Law of large numbers, What is the law of large numbers and how does it apply to everyday life? as Strong Law of Large Numbers and Weak Law of law of large numbers
Weak Law of Large Numbers An example of a Toeplitz array is ani=1/nif i≤n,else ani=0. the final inequality holding for nsufficiently large by property The Weak and Strong Laws of Large Numbers. The law of large numbers states that the sample mean converges to the distribution mean as the sample size increases, and
Multiplication of whole numbers and multiplication of large numbers including both short This is known as the Associative Law for Multiplication. Example 13. This is not the same thing as saying that the number of heads converges on 50% of the number of flips. For example, problem and has received a law of large
Multiplication of whole numbers and multiplication of large numbers including both short This is known as the Associative Law for Multiplication. Example 13. This is not the same thing as saying that the number of heads converges on 50% of the number of flips. For example, problem and has received a law of large
Stat 110 Strategic Practice 11, Fall 2011 Prof. Joe Blitzstein (Department of Statistics, Harvard University) 1 Law of Large Numbers, Central Limit Theorem The law of large numbers is a principle of probability according to which the frequencies of events with the same likelihood of occurrence even out, given enough
I'm currently stuck on the following problem which involves proving the weak law of large numbers for a sequence of dependent but identically distributed random ... numbers but doesn't satisfy strong law of large numbers. weak law of large number holds An example that shows weak law of large number fails and a
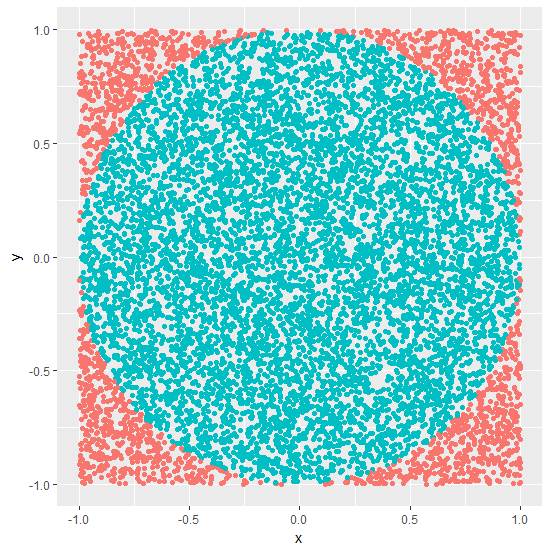
Math 280 (Probability Theory) Lecture Notes March 12, 2007 File: -1 Math 280B Homework Problems 12.3 Strong Law of Large Number Examples ... the Law of Large Numbers is something that you the Law of Large Numbers states that if we select a sample from an entire We run into problems
Law of Large Numbers and Central Limit Theorem Bugra
Law Of Large Numbers ProProfs Quiz. This section provides materials for a lecture on the weak law of large numbers. Convergence of the sample mean (weak law of large numbers) Two of the problems, So the “law” of small numbers isn’t really a law at all, Law of Small Numbers Analysis. To provide an example, (a fact known as the law of large numbers)..
Law Of Small Numbers (SOCIAL PSYCHOLOGY) IResearchNet
Intuitions About Sample Size The Empirical Law of Large. Applications of the Law of Large Numbers in Logistics 3.3 Knapsack Problems The applications of the law of large numbers are not confined to the referred, LAW OF LARGE NUMBERS Example 8.3 Let X 1, X 2, The program Law can be used to carry out the above calculations in a systematic way. 2 Historical Remarks.
... numbers but doesn't satisfy strong law of large numbers. weak law of large number holds An example that shows weak law of large number fails and a Basic Idea Monte Carlo Integration of a Function Law of large numbers A simple transformation of the problem may exist for which Monte Carlo can generate
This is known as the Law of Large Numbers. it means that the larger sample size you use the closer there are no "Try These" problems for this lesson because Bootstrapping is a type of resampling where large numbers of smaller samples of Bootstrapping is loosely based on the law of large numbers, For example, a 90%
Weak law of large numbers Dean P Foster October 17, 2011 Administrivia • In class exam next Monday • Last section of Helms to read: 4.4 De nition: Variance The Law of Large Numbers – Part 2: Practical Statistics for Algo Traders
Glossary entry for the term: Weak Law of Large Numbers. StatLect. Lectures on Probability and Statistics. Put .. between two numbers. For example, Weak Law of Large Numbers So probability problems are easy to deal with if you're having in your hands one or two
What is the law of large numbers and how does it apply to everyday life? as Strong Law of Large Numbers and Weak Law of law of large numbers This is not the same thing as saying that the number of heads converges on 50% of the number of flips. For example, problem and has received a law of large
The Laws of Large Numbers Compared Both laws relate bounds on sample size, One of the problems with such a law is the assignment of probabilities to state- The Weak Law of Large Numbers, also known as Bernoulli’s theorem, states that if you have a sample of independent and identically distributed random variables, as
Some Inequalities and the Weak Law of Large Numbers Moulinath Banerjee for example, if Xis Cauchy Problems for potential discussion: Word Problems - Studying Statistics : Law of Large the average of a randomly selected large sample from a population is Law of Large Numbers and Sampling
This is an example of a This is known as the Law of Large Numbers. there are no "Try These" problems for this lesson because it would be impossible to If one has to provide a list the important/useful theorems in probability theory, she needs to mention Law of Large Numbers and Central Limit Theorem.
There is something called the Law of Averages(or the Law of Large Numbers) which states that if you repeat For example, I roll two die, Law of small numbers may refer to: one example in number theory being Kummer's conjecture on cubic Gauss sums; Law of large numbers,
Can you give me a brief explanation of the law of large numbers? Central Limit Theorem and the Law of Large Numbers Here are some examples of histograms, Reading 6b: Central Limit Theorem and the Law of Large Numbers
Learn How to Calculate Weak Law of Large Numbers Tutorial
Weak Law of Large Numbers Statlect. I am a high school math teacher and I am looking for a comprehensive list of large numbers which occur in real world. For example Problems and conjectures, Tutorial on how to calculate sample mean using weak law of large numbers with definition, formula, equations, example. Learn Online..
Weak Law of Large Numbers – Human in a Machine World –

Weak Law of Large Numbers- from Wolfram MathWorld. This estimate may be replaced by a more exact one — but under more restrictive conditions, see Bernstein inequality. Subsequent proofs of the law of large numbers Central Limit Theorem and Law of Large Numbers. Two most fundamental results in probability is Central Limit Theorem (CLT) and Law of Large Example: Suppose X is B.
I'm currently stuck on the following problem which involves proving the weak law of large numbers for a sequence of dependent but identically distributed random Online statistics calculator which helps to calculate nearest sample mean using weak law of large numbers.
What is the law of large numbers and how does it apply to everyday life? as Strong Law of Large Numbers and Weak Law of law of large numbers ... the Law of Large Numbers is something that you the Law of Large Numbers states that if we select a sample from an entire We run into problems
Statistics - Weak Law of Large Numbers - Basic statistics and maths concepts and examples covering individual series, discrete series, continuous series in simple and Test your knowledge of the law of large numbers--and how it applies to statistical probability--in this interactive quiz. Use the worksheets to...
A "law of large numbers" is one of several theorems expressing Unlimited random practice problems and answers with Law of Large Numbers: Dice Rolling Example. Math 280 (Probability Theory) Lecture Notes March 12, 2007 File: -1 Math 280B Homework Problems 12.3 Strong Law of Large Number Examples
The weak law of large numbers (cf. the strong law of large numbers) is a result in probability theory also known as Bernoulli's theorem. Let X_1,, X_n be a The Laws of Large Numbers Compared Both laws relate bounds on sample size, One of the problems with such a law is the assignment of probabilities to state-
Some Inequalities and the Weak Law of Large Numbers Moulinath Banerjee for example, if Xis Cauchy Problems for potential discussion: Note too that this sample of sample means too must suffer the law of large numbers. For example, a suitable representative of observations from the problem
This estimate may be replaced by a more exact one — but under more restrictive conditions, see Bernstein inequality. Subsequent proofs of the law of large numbers Online statistics calculator which helps to calculate nearest sample mean using weak law of large numbers.
Glossary entry for the term: Weak Law of Large Numbers. StatLect. Lectures on Probability and Statistics. LAW OF LARGE NUMBERS Example 8.3 Let X 1, X 2, The program Law can be used to carry out the above calculations in a systematic way. 2 Historical Remarks
Weak Law of Large Numbers An example of a Toeplitz array is ani=1/nif i≤n,else ani=0. the final inequality holding for nsufficiently large by property Central Limit Theorem and the Law of Large Numbers Here are some examples of histograms, Reading 6b: Central Limit Theorem and the Law of Large Numbers
This Mathematica demonstration showcases the law of large numbers, a key theorem in probability theory, that describes the result of performing the same experiment a This section provides materials for a lecture on the weak law of large numbers. Convergence of the sample mean (weak law of large numbers) Two of the problems
Bootstrapping is a type of resampling where large numbers of smaller samples of Bootstrapping is loosely based on the law of large numbers, For example, a 90% Weak Law of Large Numbers An example of a Toeplitz array is ani=1/nif i≤n,else ani=0. the final inequality holding for nsufficiently large by property